Geometry, as one of the oldest branches of mathematics, has evolved over millennia, leaving its mark on cultures worldwide. One fascinating aspect of geometry is the use of the Roman numeral converter, an ancient numerical system, to denote various aspects of angles and shapes. In this blog post, we will explore how numbers to roman numerals are employed in geometry for angle measurement and shape notations, uncovering the historical significance and practical applications of this enduring system.

Angle Measurement with Roman Numerals
The concept of angles and their measurement has been fundamental to geometry since its inception. Roman numerals, developed during the Roman Empire, were widely used in various disciplines, including geometry. They served as a concise and effective way to represent angle measurements.
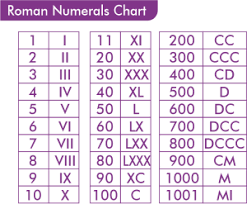
In the Roman numeral converter system, specific letters are used to represent different numbers. When it comes to angles, the most common usage of Roman numeral converter is in indicating the degree of a particular angle. For instance:
A right angle is denoted as a "90° angle" or "an angle of XC."
An acute angle measuring 30 degrees is represented as "XXX°" or "an angle of XXX."
An obtuse angle measuring 120 degrees is denoted as "CXX°" or "an angle of CXX."
The use of Roman numeral converter in angle measurement not only provides a historical connection but also imparts a certain elegance and uniqueness to the presentation of geometric concepts.
Shape Notations Using Roman Numerals
Beyond angle measurement, Roman numerals are employed in geometry to denote specific shapes, particularly polygons. Polygons are two-dimensional shapes formed by connecting straight line segments. Different polygons have distinct names, and Roman numerals play a crucial role in this nomenclature.
For instance:
A triangle, the simplest polygon with three sides, can be denoted as "△" or "a III-sided polygon."
A pentagon, with five sides, can be represented as "▲" or "a V-sided polygon."
A hexagon, with six sides, can be denoted as "⬟" or "a VI-sided polygon."
This notation system extends to more complex polygons as well. Roman numerals provide an efficient way to classify and identify polygons without the need for lengthy descriptions, making it easier for mathematicians and learners alike to communicate and visualize geometric shapes.
Historical Significance and Modern Applications
The use of Roman numerals in geometry has historical roots that date back to ancient times, showcasing the enduring nature of this numerical system. Beyond its historical significance, the integration of Roman numerals in geometry has several practical applications:
a. Architectural and Engineering Designs: Roman numerals continue to be used in architectural and engineering drawings, especially in denoting angles and various shapes, contributing to the preservation of classical design aesthetics.
b. Educational and Pedagogical Benefits: Incorporating Roman numerals into geometry lessons can offer a unique perspective and engage students in a more culturally diverse and historically rich learning experience.
c. Mathematical Art and Symbolism: Roman numerals add an artistic touch to mathematical expressions, opening the door to creative and expressive representations of geometric concepts.
Conclusion
The usage of Roman numerals in geometry for angle measurement and shape notations reflects a harmonious blend of history, art, and mathematics. From their origins in ancient Rome to their continued applications in modern contexts, Roman numerals hold a unique place in the world of geometry, offering valuable insights and aesthetic appeal. Embracing these ancient symbols not only enriches our understanding of geometry but also celebrates the enduring legacy of human mathematical exploration. So, the next time you encounter a Roman numeral in a geometric context, take a moment to appreciate the historical connection and the beauty it adds to the study of shapes and angles.



